北里大学物理2013年第2問
次の問い(問1~問6)の空所四角に入る適語を解答群から選択せよ。(解答番号15~22)
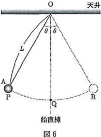
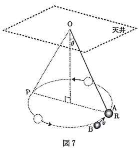
図6のように、一端を天井の点Oに固定した長さL[m]の軽い糸の他端に、質量m[kg]の小物体Aを取りつけた。糸がたるまないようにして、糸と船直線のなす角がθ[rad]となる点PでAを静かに放したところ、Aは点Oの真下の点Qを通過後、点Pと同じ高さの点Rに達した。ただし、重力加速度の大きさをg[m/s2]とし、点Qを重力による位置エネルギーの基準点とする。

- 問1 Aが点Pの位置にあるとき、Aの重力による位置エネルギーは15[J]である。
解答群- (1) mgL
- (2) mgLsinθ
- (3) mgLcosθ
- (4) mgLtanθ
- (5) mgLsinθ
- (6) mgLcosθ
- (7) mgLtanθ
- (8) mgL(1−sinθ)
- (9) mgL(1−cosθ)
- (10) mgL(1−tanθ)
- 問2 点QでのAの速さは16[m/s]である。また、このときの糸の張力の大きさは17Nである。
16の解答群- (1) √gL
- (2) √gLsinθ
- (3) √gLcosθ
- (4) √gLtanθ
- (5) √gLsinθcosθ
- (6) √gLsinθtanθ
- (7) √2gL
- (8) √2gLsinθ
- (9) √2gLcosθ
- (10) √2gLtanθ
- (11) √2gLsinθcosθ
- (12) √2gLsinθtanθ
- (13) √2gLsinθ
- (14) √2gLcosθ
- (15) √2gLtanθ
- (16) √2gL(1−sinθ)
- (17) √2gL(1−cosθ)
- (18) √2gL(1−tanθ)
- (1) mg
- (2) 2mg
- (3) 3mg
- (4) mgsinθ
- (5) mgcosθ
- (6) mgtanθ
- (7) mg(2−sinθ)
- (8) mg(2−cosθ)
- (9) mg(2−tanθ)
- (10) mgsinθ
- (11) mgcosθ
- (12) mgtanθ
- (13) mg(3−2sinθ)
- (14) mg(3−2cosθ)
- (15) mg(3−2tanθ)
- 問3 BがAに衝突した直後の、Aの速さは18[m/s]であり、Bの速さは19[m/s]である。
解答群- (1) 0
- (2) 16
- (3) 13
- (4) 12
- (5) 23
- (6) 56
- (7) 1
- (8) 76
- (9) 43
- (10) 35
- (11) 116
- (12) 2
- 問4 Aが円連動しているとき、糸の張力の大きさをm、g、θを用いて表すと20[N]である
解答群- (1) mg
- (2) 2mg
- (3) 3mg
- (4) mgsinθ
- (5) mgcosθ
- (6) mgtanθ
- (7) mg(2−sinθ)
- (8) mg(2−cosθ)
- (9) mg(2−tanθ)
- (10) mgsinθ
- (11) mgcosθ
- (12) mgtanθ
- (13) mg(3−2sinθ)
- (14) mg(3−2cosθ)
- (15) mg(3−2tanθ)
- 問5 Aが円連動していることから、Bの衝突前の速さvをg、L、θを用いて表すと21[m/s]である。
解答群- (1) √2gL
- (2) √2gLsinθ
- (3) √2gLcosθ
- (4) √2gLtanθ
- (5) √2gLsinθcosθ
- (6) √2gLsinθtanθ
- (7) 32√gL
- (8) 32√gLsinθ
- (9) 32√gLcosθ
- (10) 32√gLtanθ
- (11) 32√gLsinθcosθ
- (12) 32√gLsinθtanθ
- (13) √2gLsinθ
- (14) √2gLcosθ
- (15) √2gLtanθ
- (16) √2gL(1−sinθ)
- (17) √2gL(1−cosθ)
- (18) √2gL(1−tanθ)
- 問6 Aの円連動の周期をg、L、θを用いて表すと22[s]である。
解答群- (1) π2√Lgsinθ
- (2) π2√Lgcosθ
- (3) π2√Lg
- (4) π2√Lsinθg
- (5) π2√Lcosθg
- (6) π√Lgsinθ
- (7) π√Lgcosθ
- (8) π√Lg
- (9) π√Lsinθg
- (10) π√Lcosθg
- (11) 2π√Lgsinθ
- (12) 2π√Lgcosθ
- (13) 2π√Lg
- (14) 2π√Lsinθg
- (15) 2π√Lcosθg
つぎに、Aが点Rの位置に達した瞬間に、質量12[kg]の小物体Bを、Aの運動する鉛直面と垂直に速さv[m/s]でAと弾性衡実させたところ、図7のようにAは糸と鉛直線のなす角θ[rad]を一定に保ったまま水平面内を円運動した。